I agree with Lauren, Cara, Sarah,
and Zach that the shortesr split-line method is the best and most nonpartisan way
to redistrict. While the other methods have good intentions, they are prone to
gerrymandering; in fact one of them is exactly that! With this method, the
state is first split in half using the shortest line and this continues until
you have the desired number of districts. If there are an odd number of
districts, the process is almost identical but a bit more complicated (see
video for the exact process). Because this method is purely mathematics, there
is no argument against whether there is bias held by those in charge of drawing
the lines. In the other methods, citizens must trust people to draw unbiased
districts, but it is evident that is not possible. If someone is given the opportunity
to benefit their party they will most certainly take it. This biased line
drawing leads to corruption and one party dominating over another. Also, while
playing the redistricting game, it was difficult to keep the districts equal
while keeping specific things in mind; the short split-line method eliminates that
because it’ focus is making the districts equal. The only fault in this method
is that there can be unintentional gerrymandering. Because of the way one
district is drawn, it may favor one party over another. Ultimately, the shortest split –line method is the most effective method for redistricting. It is very
clear none of the methods are perfect, but the shortest split-line method is the
one with that is most unbiased and has the least amount of flaws. This method
will eliminate biased redistricting and equally divide the districts; exactly
the change that is needed.
This is what Texas and California would look like if the Short Split-Line method was used for redistricting!
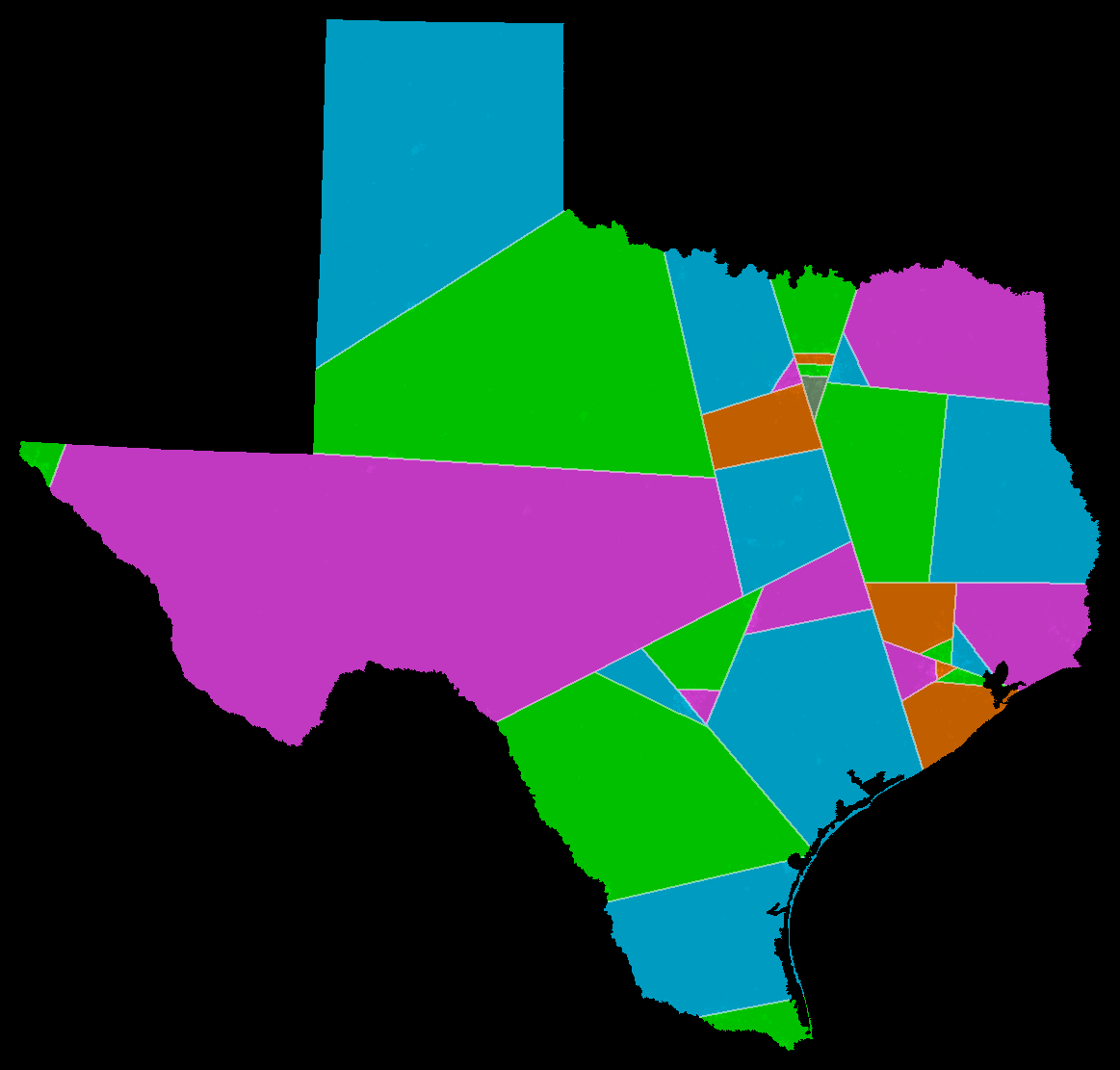

"It is very clear none of the methods are perfect, but the shortest split-line method is the one with that is most unbiased and has the least amount of flaws."
ReplyDeleteWould it be better to change our electoral system all together?